by Michael Liss
We might have been a free and a great people together, but a communication of grandeur & of freedom it seems is below their dignity. —Thomas Jefferson, “Jefferson Draft” of the Declaration of Independence, 1776.

George Washington may have been the “Indispensable Man” whose strength we used as our North Star, Benjamin Franklin the cherubic, ever optimistic face we showed to the outside world, James Madison the primary architect of our Constitution, but, for raw emotion wedded to soaring eloquence, no other American of the Revolutionary period quite approached Thomas Jefferson.
I have never been a big Jefferson fan. He runs a little hot for my taste. I prefer the brooding-yet cerebral miniaturist approach of Lincoln, who says, “We are not enemies, but friends. We must not be enemies. Though passion may have strained, it must not break our bonds of affection.”
My passion right now is somewhat strained, and I’m concerned that being a free and great people together might be a bit far-fetched. Like just about everyone else on my side of the divide, I want to know what happened and why. How on Earth did we manage to break the GPS and end up right back where we started?
Let us take a deep breath, and, with the cool professionalism of the Federal Railroad Administration’s Accident Management Branch, examine the wreck. Yes, I know, Trump has targeted the Agency and plans to roll back safety regulations on hazardous gas transit, crew member requirements and fatigue risk, but this is only December 2024, and we are still living the good life.
OK, exhale.
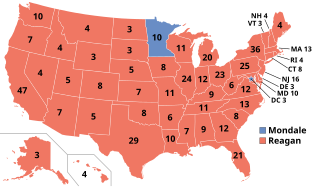
This was not a landslide, not actually, and not by any historical metric. Harris clearly lost, Trump clearly won, but Trump’s popular and Electoral Vote margins are more in sync with the trench-warfare-limited-gains of the non-Obama elections we’ve had since 2000. Similarly, it was not a mandate for anything, no matter how often Trump and his team in Congress and the media claim that.
Why does this feel like a landslide, when historical context tells us it isn’t anywhere near one? Read more »

 My friend R is a man who takes his simple pleasures seriously, so I asked him to name one for me. Boathouses, he said, without hesitation.
My friend R is a man who takes his simple pleasures seriously, so I asked him to name one for me. Boathouses, he said, without hesitation.

 Early on, Magona presents readers of Beauty’s Gift with a startling image: the beautiful and ‘beloved’ Beauty laid to rest in an opulent casket, which is then fixed in the earth with cement to prevent theft. Her friends’ memories of Beauty’s charisma and kindness are concretized by the weight of her death from AIDS. From the outset, funerals emerge not merely as a plot point but a structuring device for understanding the social and political implications of the AIDS crisis in South Africa. After opening her novel with Beauty’s funeral, Magona continues with vignettes about various stages of illness, death, and grief. These include a wake, the mourning period, Beauty’s posthumous
Early on, Magona presents readers of Beauty’s Gift with a startling image: the beautiful and ‘beloved’ Beauty laid to rest in an opulent casket, which is then fixed in the earth with cement to prevent theft. Her friends’ memories of Beauty’s charisma and kindness are concretized by the weight of her death from AIDS. From the outset, funerals emerge not merely as a plot point but a structuring device for understanding the social and political implications of the AIDS crisis in South Africa. After opening her novel with Beauty’s funeral, Magona continues with vignettes about various stages of illness, death, and grief. These include a wake, the mourning period, Beauty’s posthumous 
 Humans are beings of staggering complexity. We don’t just consist of ourselves: billions of bacteria in our gut help with everything from digestion to immune response.
Humans are beings of staggering complexity. We don’t just consist of ourselves: billions of bacteria in our gut help with everything from digestion to immune response.

 Sughra Raza. Self Portrait Against Table Mountain. August, 2019.
Sughra Raza. Self Portrait Against Table Mountain. August, 2019.
 Much philosophical writing about food has included discussions of whether and why food can be a serious aesthetic object, in some cases aspiring to the level of art. These questions often turn on whether we create mental representations of flavors and textures that are as orderly and precise as the representations we form of visual objects.
Much philosophical writing about food has included discussions of whether and why food can be a serious aesthetic object, in some cases aspiring to the level of art. These questions often turn on whether we create mental representations of flavors and textures that are as orderly and precise as the representations we form of visual objects.