Tag: quantum mechanics

Bouncing Droplets Refute the Multiverse?

Posted on by David Kordahl

by David Kordahl

There’s an old story, popularized by the mathematician Augustus De Morgan (1806-1871) in A Budget of Paradoxes, about a visit of Denis Diderot to the court of Catherine the Great. In the story, the Empress’s circle had heard enough of Diderot’s atheism, and came up with a plan to shut him up. De Morgan writes,

Diderot was informed that a learned mathematician was in possession of an algebraical demonstration of the existence of God, and would give it him before all the Court, if he desired to hear it. Diderot gladly consented: though the name of the mathematician is not given, it was Euler. He advanced towards Diderot, and said gravely, and in a tone of perfect conviction: Monsieur, (a + bn) / n = x, donc Dieu existe; répondez! Diderot, to whom algebra was Hebrew, was embarrassed and disconcerted; while peals of laughter rose on all sides. He asked permission to return to France at once, which was granted.

De Morgan concedes that the story may not be true, yet even at face value, it’s a puzzling anecdote. De Morgan tells us that “Euler was a believer in God, downright and straightforward.” It’s obvious that an algebraic expression has no bearing on God’s existence. In the story, Diderot misses that point (the historical Diderot was not, in fact, so ignorant), but it leads the modern reader to wonder…so what? Diderot may or may not have been an overly dogmatic atheist, but should a “downright and straightforward” believer really defend his beliefs with bullshit?

The modern reader might then ask a followup question. What questionable claims today have been made harder to dismiss by cloaking them in math?

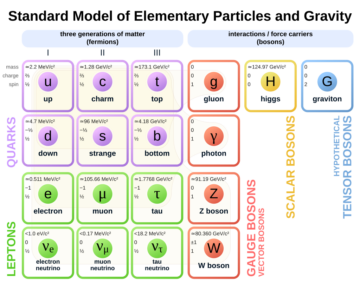
A few possibilities from economics and psychology come to mind, but this column will discuss a new book that advances the charge against quantum physics. Escape from Shadow Physics: The Quest to End the Dark Ages of Quantum Theory, by Adam Forrest Kay, forwards some complaints that readers of popular physics may find find a little familiar (cf. Sabine Hossenfelder’s Lost in Math, or Adam Becker’s What Is Real?), but Kay introduces enough historical and philosophical breadth to make his version my new favorite of the bunch, if with some reservations about its conclusions. Read more »



Thursday, September 19, 2024

Wheels Within Wheels: The Hopf Fibration And Physics II

Posted on by Jochen Szangolies

by Jochen Szangolies

Crucial step in the proof that 1 + 1 = 2, coming on page 379 of Russell and Whitehead’s formalist tour-de-force Principia Mathematica.

In the last column, I have argued against the idea that understanding in mathematics and physics is transmitted via genius leaps of insight into obscure texts rife with definitions and abstract symbols. Rather, it is more like learning to cook: even if you have memorized the cookbook, your first soufflé might well fall in on itself. You need to experiment a little, get a feel for ingredients, temperatures, resting times and the way they interact before you get things right. Or take learning to ride a bike: the best textbook instructions won’t keep you from skinning your knees on your first try.

Practical skills are acquired through practice, and doing maths is just such a skill. However, mathematics (and physics by extension) may be unique in that it likes to pretend otherwise: that understanding is gleaned from definitions; that the manipulation of symbols on a page according to fixed rules is all there is to it. But no: just as you need an internal, intuitive model of yourself on a bike, its reactions to shifts in weight and ways to counteract developing instabilities, the skilled mathematician has an intuition of the mathematical objects under their study, and only later is that intuition cast into definitions and theorems.

At the risk of digressing too far, this is a general feature of human thought: we always start with an intuitive conception, only to later dress it up in formal garb to parade it before the judgment of others. We are not logical, but ‘analogical’ beings, our thoughts progressing as a series of dimly-grasped associations rather than crisp step-by-step derivations. If we do find ourselves engaged in the latter, then as a laborious, explicit, and slow ‘System 2’-exercise, rather than the intuitive leaps of ‘System 1’.

Indeed, it couldn’t be otherwise: how should we know whether a definition is accurate, if we didn’t have a grasp on the concept beyond that definition? Read more »

Tuesday, August 20, 2024

Music Of The Spheres: The Hopf Fibration And Physics

Posted on by Jochen Szangolies

by Jochen Szangolies

The particles of the Standard Model (and gravity). Image credit: Cush, CC0, via Wikimedia Commons

Modern physics in its full mathematical splendor introduces an array of unfamiliar concepts that daunt the initiate, and often even bewilder the pro (or is that just me?). A part of it is just that it’s a complex topic, and its objects of study are far removed from everyday experience: a quark or a black hole or a glueball is not something you’re likely to find on your desk. Well, maybe the latter, if you’ve been sloppy while crafting recently, but as so very often, physicists further confuse things by giving familiar names to unfamiliar concepts (spin, I’m looking your way).

But saying ‘it’s complicated’ is merely a fig leaf. Lots of things are complicated, and we manage to navigate them with ease. Many jobs involve reams of specialist knowledge, from plumbing to hedge-fond management, and even just navigating our webs of social relationships comes with considerable overhead. So what is it that makes physics special?

There is, of course, the already mentioned issue of the remoteness of its central concepts. Many of the complicated tasks we solve are so ingrained to us that we scarcely notice their complexity—the act of throwing a ball, or catching it out of thin air in flight, involves calculations that, in a realistic setting, stymied the efforts of robotics engineers for a long time. Likewise, the acquisition of language—even present-day Large Language Models (LLMs) still need to ‘read’ tens of trillions of words to acquire a degree of language fluency a human child can pick up just from what is spoken around them in their first couple of years. By comparison, an average reader would take something like 80.000 years of continuous reading time to ingest the text on which an LLM is trained!

These are tasks that, in some manner, are performed ‘natively’ by the human brain, without us noticing their complexity. Such tasks are sometimes classed as ‘System 1’-tasks in the dual-system psychology popularized by Daniel Kahnemann in his bestselling popular science book Thinking, Fast and Slow. In contrast, solving a mathematical equation or reasoning through a logic puzzle are step-by-step, explicit ‘System 2’-tasks you have to concentrate on—they’re not performed ‘by themselves’ the way catching a ball is. Read more »

Monday, April 22, 2024

Physical Analogies and Field Theory

Posted on by David Kordahl

by David Kordahl

In popular media, physics often comes up for one of two competing reasons. The first is to introduce a touch of mysticism without labeling it as such. Whether it’s Carl Sagan talking about our bones as stardust, or Lisa Randall suggesting some extra dimensions of space, these pronouncements are often presented to evoke the listener’s primal awe—an ancient and venerable form of entertainment. The second reason is just as venerable, and often as entertaining. Sometimes, physics just gets results. Think of MacGuyver in MacGuyver, Mark Watney in The Martian, or those stunt coordinators in Mythbusters—characters whose essential pragmatism couldn’t be further from the tremulous epiphanies of the theorists.

Dramatically, the esoteric and the everyday can seem like opposites, and many fictional plots seem to advise against bringing them together. Mad scientists, those cautionary anti-heroes like Drs. Frankenstein and Manhattan, are often characters who both stumble upon hidden truths and put them to terrible use. But in the real world of physics, it’s common to forge connections between the realms.

Physical analogies, examples that link unfamiliar physics to everyday experience, are important in forging such connections. Waves in an Impossible Sea: How Everyday Life Emerges from the Cosmic Ocean, a new book by the physicist Matt Strassler, is an impressive attempt to explain contemporary physics using little math but many analogies. Strassler mainly goes against the archetype of the theoretical physicist as the purveyor of primal awe. Instead, he’s a practiced teacher, more interested in accuracy than amazement. In seven concise sections—Motion, Mass, Waves, Fields, Quantum, Higgs, and Cosmos—he covers the basics of physics with minimal fuss, but with a charmingly dorky earnestness. Read more »

Monday, November 13, 2023

Münchhausen And The Quantum: Dragging Ourselves Out Of The Swamp

Posted on by Jochen Szangolies

by Jochen Szangolies

Münchhausen dragging himself out of the swamp. Image credit: public domain.

There seems no obvious link between tall war-tales, shared among a circle of German aristocrats in the 1760s, and quantum mechanics. The former would eventually come to form the basis of the exploits of Baron Münchhausen, the partly fictionalized avatar of Hieronymus Karl Friedrich, Freiherr von Münchhausen, famous for his extravagant narratives, while the latter is the familiar, yet vexingly incomprehensible, theory of the ‘microscopic’ realm developed more than 150 years later. Both, however, seem to equally beggar belief: which is stranger—riding a cannonball across a battlefield (and back), or seemingly being in two places at once? Reconnecting a horse bisected by a falling gate, or deciding the fate of a both-dead-and-alive cat by opening a box?

But beyond mere bafflement, the stories of Münchhausen’s exploits have inspired a philosophical conundrum relevant to the question of quantum reality. Perhaps the Lügenbaron’s most famous story, it concerns his getting trapped in the swamp on his horse, a conundrum which is solved by pulling the both of them out by his own plait of hair.

The power of this image was appreciated by Friedrich Nietzsche, who in Beyond Good and Evil likened the concept of ‘free will’ to being a causa sui, “with a courage greater than Munchhausen’s, pulling yourself by the hair from the swamp of nothingness up into existence”. But in its most famous formulation, due to the German philosopher Hans Albert, who died last week at the venerable age of 102, it comes in the form of the Münchhausen-trilemma. Any attempt at finding a final justification, according to Albert, must end in either of the following options:

  • Infinite regress: whatever is supposed to yield this justification must be justified itself (turtles all the way down)

  • Circularity: the justification of some proposition presumes that very proposition’s truth (the turtle stands on itself)

  • Dogma: the regress is artificially broken by postulating a ‘buck-stops-here’ justification that is assumed itself unassailable and without need for further explanation (the final turtle is supported by nothing)

Each of the above seems to frustrate any attempt at finding any sort of certain ground to stand on—and, lacking Münchhausen’s ability to drag ourselves out by our own hair, sees us firmly bogged down in the mire of uncertainty. An infinite regress will never reach its conclusion, thus, like a parent frustrated with an endless series of ‘Why?’, we may be tempted to cut it short by an imagined regress-stopper—‘because I/God/the laws of physics say so’, but just because the journey stops, doesn’t mean we’ve arrived at our destination.

What can be done in the face of the trilemma? Read more »

Monday, July 17, 2023

How Quantum Models Work

Posted on by David Kordahl

by David Kordahl

A notable theorist visits a notable laboratory (Stephen Hawking at CERN, 2013)

The science lab and the theory suite

If you spend any time doing science, you might notice that some things change when you close the door to the lab and walk into the theory suite.

In the laboratory, surprising things happen, no doubt about it. Depending on the type of lab you’re working in, you might see liquid nitrogen boiling out from a container, solutions changing color only near their surfaces, or microorganisms unexpectedly mutating. But once roughly the same thing happens a few times in a row, the conventional scientific attitude is to suppose that you can make sense of these observations. Sure, you can still expect a few outliers that don’t follow the usual trends, but there’s nothing in the laboratory that forces one to take any strong metaphysical positions. The surprises, instead, are of the sort that might lead someone to ask, Can I see that again? What conditions would allow this surprise to reoccur?

Of course, the ideas discussed back in the theory suite are, in some indirect way, just codified responses to old observational surprises. But scientists—at least, young scientists—rarely think in such pragmatic terms. Most young scientists are cradle realists, and start out with the impression that there is quite a cozy relationship between the entities they invoke in the theory suite and the observations they make back in the lab. This can be quite confusing, since connecting theory to observation is rarely so straightforward as simply calculating from first principles.

The types of experiments I’ve had been able to observe most closely involve electron microscopes. For many cases where electron microscopes are involved, workers will use quantum models to describe the observations. I’ve written about quantum models a few times before, but I haven’t discussed much about how quantum physics models differ from their classical physics counterparts. Last summer, I worked out a simple, concrete example in detail, and this column will discuss the upshot of that, leaving out the details. If you’ve ever wondered, how exactly do quantum models work?—or even if you haven’t wondered, but are wondering now that I mention it—well, read on. Read more »

Monday, April 3, 2023

Gödel’s Proof and Einstein’s Dice: Undecidability in Mathematics and Physics – Part III

Posted on by Jochen Szangolies

by Jochen Szangolies

There are countless virtual realities, albeit as of yet, not exactly a replacement for the real thing. Image credit: wikimedia commons.

The simulation argument, most notably associated with the philosopher Nick Bostrom, asserts that given reasonable premises, the world we see around us is very likely not, in fact, the real world, but a simulation run on unfathomably powerful supercomputers. In a nutshell, the argument is that if humanity lives long enough to acquire the powers to perform such simulations, and if there is any interest in doing so at all—both reasonably plausible, given the fact that we’re in effect doing such simulations on the small scale millions of times per day—then the simulated realities greatly outnumber the ‘real’ realities (of which there is only one, barring multiversal shenanigans), and hence, every sentient being should expect their word to be simulated rather than real with overwhelming likelihood.

On the face of it, this idea seems like so many skeptical hypotheses, from Cartesian demons to brains in vats. But these claims occupy a kind of epistemic no man’s land: there may be no way to disprove them, but there is also no particular reason to believe them. One can thus quite rationally remain indifferent regarding them.

But Bostrom’s claim has teeth: if the reasoning is sound, then in fact, we do have compelling reasons to believe it to be true; hence, we ought to either accept it, or find flaw with it. Luckily, I believe that there is indeed good reason to reject the argument. Read more »

Monday, March 27, 2023

Quantum Field Theory, “Easier Than Easy”

Posted on by David Kordahl

by David Kordahl

The book under review.

I began reading Anthony Zee’s most famous book, Quantum Field Theory in a Nutshell, at Muncher’s Bakery in Lawrence, Kansas, where, as a would-be quantum field theorist in 2010, Zee’s book taught me to evaluate Gaussian integrals. Zee made it all seem almost trivial, but his fast style belied the true expectation that his book would be read slowly, pen in hand, the reader studiously working their way from one line to the next. You couldn’t escape the sense that Zee was a very clever man, if not a very sympathetic teacher. This was a book whose readers would select it. If they couldn’t proceed, well, who was really to blame?

I never did become a quantum field theorist, though that’s hardly Zee’s fault. (At that point, I barely had the patience to sit and eat a donut.) Thankfully, Zee has now published an even swifter book, Quantum Field Theory, As Simply as Possible, which readers of this column will be happy to know I actually finished.

On the first page, Zee comments wryly that popular physics books jumped straight from quantum mechanics to string theory—so this book fills the quantum field theory gap. Now, if you are not a physicist, you may not know what quantum field theory is. This review is for you. Unfortunately, Zee’s new book probably isn’t. For whom then, is QFT, as Simply as Possible (henceforth: QFT, ASAP) written? My own answer is that it’s perfect for a past version of myself, just way too late for that bakery. Read more »

Monday, March 6, 2023

Gödel’s Proof and Einstein’s Dice: Undecidability in Mathematics and Physics – Part II

Posted on by Jochen Szangolies

by Jochen Szangolies

Commemorative plaque at Gödel’s former house in Vienna. Image credit: By Beckerhermann – Own work, CC BY-SA 4.0, via wikimedia commons

The previous column left us with the tantalizing possibility of connecting Gödelian undecidability to quantum mechanical indeterminacy. At this point, however, we need to step back a little.

Gödel’s result inhabits the rarefied realm of mathematical logic, with its crisply stated axioms and crystalline, immutable truths. It is not at all clear whether it should have any counterpart in the world of physics, where ultimately, experiment trumps pure reason.

However, there is a broad correspondence between physical and mathematical systems: in each case, we start with some information—the axioms or the initial state—apply a certain transformation—drawing inferences or evolving the system in time—and end up with new information—the theorem to be proved, or the system’s final state. An analogy to undecidability then would be an endpoint that can’t be reached—a theorem that can’t be proven, or a cat whose fate remains uncertain.

Perhaps this way of putting it looks familiar: there is another class of systems that obeys this general structure, and which were indeed the first point of contact of undecidability with the real world—namely, computers. A computer takes initial data (an input), performs a transformation (executes a program), and produces a result (the output). Moreover, computers are physical devices: concrete machines carrying out computations. And as it turns out, there exists questions about these devices that are undecidable. Read more »

Gödel’s Proof and Einstein’s Dice: Undecidability in Mathematics and Physics – Part I

Posted on by Jochen Szangolies

by Jochen Szangolies

God’s dice? Image by S L on Unsplash

TWA Flight 702 left New York at 7 AM on Monday, Feb. 4 1974, to arrive in London at 7 PM—some 40 minutes early. We know this thanks to the meticulous note-taking habits of visionary physicist John Archibald Wheeler, coiner of such colorful terms as ‘quantum foam’, ‘wormhole’, ‘superspace’ and ‘black hole’.

Wheeler spent the flight occupied with what he is perhaps best remembered for: pondering his ‘Really Big Questions’ (RBQs), among which we find perennial mysteries such as ‘How come existence?’ or ‘What makes meaning?’. The RBQ that occupied Wheeler on this particular day, however, was one that in many ways lay at the nexus of his thought: ‘Why the quantum?’

Wheeler had been a student of Bohr and Einstein, and thus, had learned about quantum mechanics straight from the horse’s mouth. Yet, he would struggle with the implications of the theory for the rest of his life, referring to the fundamental indefiniteness of its phenomena as the ‘great smoky dragon’. He was searching for a way to dispel the smoke, and in the note composed on Flight 702, draws a surprising connection to another remote frontier of human understanding—the phenomenon of mathematical undecidability, as discovered in 1931 by the then-25 year old logician Kurt Gödel. (The note itself is available online at the John Archibald Wheeler Archive curated by Baruch Garcia.)

At first sight, one might suppose this connection to be little more than a kind of ‘parsimony of mystery’: in substituting one riddle for another, the total number of unknowns is reduced. Indeed, the idea of ripping Gödel’s result from the austere domain of mathematical logic and injecting it into physical theory is controversial—earlier, during the writing of his magisterial textbook on General Relativity, Gravitation, with Charles Misner and Kip Thorne, Wheeler had confronted Gödel himself with the idea, who did not react too enthusiastically. Read more »

Monday, October 17, 2022

Bell’s Theorem: A Nobel Prize For Metaphysics

Posted on by Jochen Szangolies

by Jochen Szangolies

Bells Theorem Crescent in Belfast, John Bell’s birth town.

There has been no shortage of articles on this year’s physics Nobel, which, just in case you’ve been living under a rock, was awarded to Alain Aspect, John Clauser, and Anton Zeilinger “for experiments with entangled photons, establishing the violation of Bell inequalities and pioneering quantum information science”. Why, then, add more to the pile?

A justification is given be John Bell himself in his 1966 review article On the Problem of Hidden Variables in Quantum Mechanics: “[l]ike all authors of noncommissioned reviews [the writer] thinks that he can restate the position with such clarity and simplicity that all previous discussions will be eclipsed”. While I like to think that I’m generally more modest in my ambitions than Bell semi-seriously positions himself here, I feel that there is a lacuna in most of the recent coverage that ought to be addressed. That omission is that while there is much talk about what the prize-winning research implies—from the possibility of groundbreaking new quantum technologies to the refutation of dearly held assumptions about physical reality—there is considerably less talk about what it, and Bell’s theorem specifically, actually is, and why it has had enough impact beyond the scientific world to warrant the unique (to the best of my knowledge) distinction of having a street named after it.

In part, this is certainly owed to the constraints of writing for an audience with a diverse background, and the fear of alienating one’s readers by delving too deeply into what might seem like overly technical matters. Luckily (or not), I have no such scruples. However, I—perhaps foolishly—believe that there is a way to get the essential content of Bell’s theorem across without breaking out its full machinery. Indeed, the bare statement of his result is quite simple. At its core, what Bell did was to derive an inequality—a bound on the magnitude of a certain quantity—such that, when it holds, we can write down a joint probability distribution for the possible values of the inputs of the inequality, where these ‘inputs’ are given by measurement results.

Now let’s unpack what this means. Read more »

Monday, May 2, 2022

The Mind and the Quantum: Complementary Perspectives

Posted on by Jochen Szangolies

by Jochen Szangolies

Figure 1: Result of asking the NightCafe-AI to draw ‘quantum consciousness’: all of the bits and pieces seem evocative and intriguing, but fail to assemble into a coherent whole. A taste of things to come?

Reading the words ‘mind’ and ‘quantum’ in close proximity on the internet rarely inspires great confidence. Indeed, all too often, all this indicates is that you’re about a click away from learning about life-changing techniques of ‘Quantum Jumping’ to a parallel reality where all your wishes come true, using ‘Quantum Healing’ to ‘holistically’ heal the ‘bodymind’, and other What the Bleep Do We Know!?-esque nonsense. Therefore, in writing about the fraught intersection of quantum mechanics and the study of consciousness, one is faced first with the challenge of convincing the reader that there is actually something of value to be gained from investing their attentional resources. So let me first consider arguments against the relevance of quantum mechanics for consciousness.

First, of course, mere woo-by-association does not make a good argument. That people have misused the buzzwords of ‘quantum’, ‘mind’, ‘consciousness’ and the like to promote shoddy self-help strategies does not entail that they can’t be combined in a sensible, and ideally even illuminating, way. But there are commonly-cited objections that deserve serious consideration.

The most common is the ‘large, warm, and wet’-argument. Quantum mechanics is often (if somewhat misleadingly) considered to be the ‘science of the small’, whose effects are typically observed at length scales far removed from everyday sizes, and thus, from brains. Furthermore, quantum effects typically requires systems to be well-isolated from the environment, to not fall prey to decoherence effects. But brains aren’t generally thus isolated—indeed, in a sense, it’s the very point of a brain to interact with the environment. Finally, quantum systems, to limit interaction, often have to be cooled down to a few degrees above absolute zero, something that again isn’t conducive to the proper functioning of a brain. Read more »

Monday, April 25, 2022

Scientific Models and Individual Experience

Posted on by David Kordahl

by David Kordahl

I’ll start this column with an over-generalization. Speaking roughly, scientific models can be classed into two categories: mechanical models, and actuarial models. Engineers and physical scientists tend to favor mechanical models, where the root causes of various effects are specified by their formalism. Predictable inputs, in such models, lead to predictable outputs. Biologists and social scientists, on the other hand, tend to favor actuarial models, which can move from measurements to inferences without positing secret causes along the way. By calling these latter models “actuarial,” I’m encouraging readers to think of the tabulations of insurance analysts, who have learned to appreciate that individuals may be unpredictable, even as they follow predictable patterns in the aggregate.

Operationally, these categories refer to different scientific practices. What I’ve called a difference between mechanical vs. actuarial models could just as well be sketched as a difference between theory-driven vs. data-driven models. Both strains have coexisted in science for the past few centuries.

Just for fun, we might attempt to caricature the history of modern science in the mechanical vs. actuarial terms introduced above. In the seventeenth century, Isaac Newton proposed a law of universal gravitation, applicable everywhere throughout the universe, which allowed naturalists to imagine that all physical effects, everywhere and for all time, were caused by physical laws, just waiting to be discovered. This view was developed to its philosophical extreme in the eighteenth century by the French mathematician, Pierre Laplace, who imagined that the universe at any particular moment implicitly contained the specifications for its entire past and future.

But in the nineteenth century, Charles Darwin introduced his theory of natural selection, which allowed naturalists to take actuarial models more seriously. Just as hidden order could cause the appearance of randomness, hidden randomness could cause the appearance of order. Read more »

Monday, September 20, 2021

Incoherent Incoherence: Freedom In A Physical World II

Posted on by Jochen Szangolies

by Jochen Szangolies

Figure 1: Statue of Ibn Rushd, author of the Incoherence of the Incoherence, in Córdoba, Spain. Image credit: Saleemzohaib, CC0, via Wikimedia Commons.

The Incoherence of the Philosophers (Tahâfut al-falâsifa) is an attempt by 11th century Sunni theologian and mystic al-Ghazâlî to refute the doctrines of philosophers such as Ibn Sina (often latinized Avicenna) or al-Fârâbî (Alpharabius), which he viewed as heretical for favoring Greek philosophy over the tenets of Islam. Al-Ghazâlî’s methodological principle was that in order to refute the assertions of the philosophers, one must first be well versed in their ideas; indeed, another work of his, Doctrines of the Philosophers (Maqāsid al-Falāsifa), gives a comprehensive survey of the Neoplatonic philosophy he sought to refute in the Incoherence.

The Incoherence, besides its other qualities, is noteworthy in that it is now regarded as a landmark work in philosophy itself. Ibn Rushd (Averroes), in response, penned the Incoherence of the Incoherence (Tahāfut al-Tahāfut), a turning point away from Neoplatonism to Aristotelianism.

In modern times, most allegations of ‘incoherence’ levied against philosophy come not from the direction of religion, but rather, from scientists’ allegations that their discipline has made philosophy redundant, supplanting it by a better set of tools to investigate the world. The perhaps most well-known example of this is Stephen Hawking’s infamous assertion that ‘philosophy is dead’, but similar sentiments are readily found. While the proponents of such allegations have not always shown shown al-Ghazâlî’s methodological scrupulousness in engaging with the body of thought they seek to refute, these are still weighty charges by some of the leading intellectuals of the day. Read more »

Monday, March 8, 2021

Hidden Worlds: Science, Truth, and Quantum Mechanics

Posted on by Jochen Szangolies

by Jochen Szangolies

Figure 1: A typical result of googling the word ‘quantum’: pretty, but not especially enlightening.

Hearing the words ‘quantum mechanics’ usually invokes images of the impossibly tiny and fleeting, phenomena just barely on the edge of existence, unfathomably far removed from everyday experience. Perhaps illustrated in the form of bright, jittery sparkly things jumping about in a PBS documentary, perhaps as amorphous, hovering blobs of improbability, perhaps, sometimes, by the confounding notion of a cat that’s somehow both dead and alive, yet neither of those.

This does the subject a disservice. It paints a picture of quantum mechanics as far removed from everyday experience, as something we need not worry about in everyday life, something for boffins in lab-coats to contend with in their arcane ways. Yet, we’re told of the fantastic properties of the quantum world: particles that can be in two places at once, or spontaneously erupt out of sheer nothingness; that can jump through walls and communicate with one another across great distances instantly; that seem to know when they’re being watched; that are somehow both wave and particle; and so on.

Quantum reality, then, is at once beyond our grasp and, apparently, a source of fantastical properties. This combination has always marked the arena of the mystical: something just out of reach, something fundamentally unknowable, that, nevertheless, holds the promise of opening the doors to a strange, new world—to powers far beyond those the mundane world holds in store. The quantum world is a hidden world, and, like other hidden worlds throughout history, access to it becomes a coveted resource—to the profit of those purporting to be able to grant it. Read more »

Monday, July 6, 2020

Reality Has Left The Building

Posted on by Thomas O'Dwyer

by Thomas O’Dwyer

Our world (made of atoms) is crammed with paradoxes. Particles act like waves, waves like particles And your cat can be dead and alive at the same time. Just step through your looking glass and welcome to the quantum world. “If you think you understand quantum mechanics, you haven’t understood quantum mechanics,” the physicist Richard Feynman once said. Of course, the non-scientific reader may respond, “Why would I want to understand it?” If a genius like Feynman became lost in the twisting labyrinth of the quantum world, abandon hope all ye who expect to become enlightened here.

Schrödinger's cat: Every event is a branch point. The cat is both alive and dead but the "alive" and "dead" cats are in different branches of the universe that are equally real but cannot interact with each other. (Wikipedia)
Schrödinger’s cat: Every event is a branch point. The cat is both alive and dead but the “alive” and “dead” cats are in different branches of the universe that are equally real but cannot interact with each other. (Wikipedia)

Quantum theory is famously opaque, and it drew dismissive grumbles from Albert Einstein. He was one of many superior minds who worried that science was abandoning its high road of rigorous clarity to dabble again in the murkiness of faith and superstition by even pondering the notion of quantum reality. Alive-dead animals, parallel universes, the existence of all times past present and future? These were for April 1 spoofs, right guys? Yet, whether one is aware of it or not, quantum mechanics has given us lasers, smartphones and many esoteric electronic components, like tunnelling diodes, from which we build our devices. They come with a weird label that says, we made them, and they work, but we don’t quite know how. Quantum computers will soon solve problems well beyond the reach of present-day digital machines – complex chemical analyses, dynamic biological processes. These will be of use to the pharmaceutical industries, and they will also model complex systems like financial transactions and climate changes. Read more »

Monday, September 2, 2019

Spooky factions at a distance

Posted on by Ashutosh Jogalekar

by Ashutosh Jogalekar

For me, a highlight of an otherwise ill-spent youth was reading mathematician John Casti’s fantastic book “Paradigms Lost“. The book came out in the late 1980s and was gifted to my father who was a professor of economics by an adoring student. Its sheer range and humor had me gripped from the first page. Its format is very unique – Casti presents six “big questions” of science in the form of a courtroom trial, advocating arguments for the prosecution and the defense. He then steps in as jury to come down on one side or another. The big questions Casti examines are multidisciplinary and range from the origin of life to the nature/nurture controversy to extraterrestrial intelligence to, finally, the meaning of reality as seen through the lens of the foundations of quantum theory. Surprisingly, Casti himself comes down on the side of the so-called many worlds interpretation (MWI) of quantum theory, and ever since I read “Paradigms Lost” I have been fascinated by this analysis.

So it was with pleasure and interest that I came across Sean Carroll’s book that also comes down on the side of the many worlds interpretation. The MWI goes back to the very invention of quantum theory by pioneering physicists like Niels Bohr, Werner Heisenberg and Erwin Schrödinger. As exemplified by Heisenberg’s famous uncertainty principle, quantum theory signaled a striking break with reality by demonstrating that one can only talk about the world only probabilistically. Contrary to common belief, this does not mean that there is no precision in the predictions of quantum mechanics – it’s in fact the most accurate scientific framework known to science, with theory and experiment agreeing to several decimal places – but rather that there is a natural limit and fuzziness in how accurately we can describe reality. As Bohr put it, “physics does not describe reality; it describes reality as subjected to our measuring instruments and observations.” This is actually a reasonable view – what we see through a microscope and telescope obviously depends on the features of that particular microscope or telescope – but quantum theory went further, showing that the uncertainty in the behavior of the subatomic world is an inherent feature of the natural world, one that doesn’t simply come about because of uncertainty in experimental observations or instrument error. Read more »

Monday, September 4, 2017

Heisenberg on Helgoland

Posted on by Ashutosh Jogalekar

by Ashutosh Jogalekar

Helgoland_Vogelperspektive_BW_2The sun was setting on a cloudless sky, the gulls screeching in the distance. The air was bracing and clear. Land rose from the blue ocean, a vague apparition on the horizon.

He breathed the elixir of pure evening air in and heaved a sigh of relief. This would help the godforsaken hay fever which had plagued him like a demon for the last four days. It had necessitated a trip away from the mainland to this tiny outcrop of flaming red rock out in the North Sea. Here he could be free not just of the hay fever but of his mentor, Niels Bohr. Perched on the rock, he looked out into the blue expanse.

For the last several months, Bohr had followed him like a shadow, an affliction that seemed almost as bad as the hay fever. It had all started about a year earlier, but really, it started when he was a child. His father, an erudite scholar but unsparing disciplinarian, made his brother and him compete mercilessly with each other. Even now he was not on the best terms with his brother, but the cutthroat competition produced at least one happy outcome: a passion for mathematics and physics that continued to provide him with intense pleasure.

He remembered those war torn years when Germany seemed to be on the brink of collapse, when one revolution after another threatened to tear apart the fabric of society. Physics was the one refuge. It sustained him then, and it promised to sustain him now.

If only he could understand what Bohr wanted. Bohr was not his first mentor. That place of pride belonged to Arnold Sommerfeld in Munich. Sommerfeld, the man with the impeccably waxed mustache who his friend Pauli called a Hussar officer. Sommerfeld, who would immerse his students not only in the latest physics but in his own home, where discussions went on late into the night. Discussions in which physics, politics and philosophy co-existed. His own father was often distant; Sommerfeld was the father figure in his life. It was also in Sommerfeld’s classes that he met his first real friend – Wolfgang Pauli. Pauli was still having trouble attending classes in the morning when there were all those clubs and parties to frequent at night. He always enjoyed long discussions with Pauli, the ones during which his friend often complimented him by telling him he was not completely stupid. It was Pauli who had steered him away from relativity and toward the most exciting new field in physics – quantum theory.

Read more »

Monday, July 10, 2017

Black Holes and the Curse of Beauty: When Revolutionary Physicists Turn Conservative

Posted on by Ashutosh Jogalekar

by Ashutosh Jogalekar

Main-qimg-da0bd0564345ac4af20890fb6dc10820-cOn September 1, 1939, the leading journal of physics in the United States, Physical Review, carried two remarkable papers. One was by a young professor of physics at Princeton University named John Wheeler and his mentor Niels Bohr. The other was by a young postdoctoral fellow at the University of California, Berkeley, Hartland Snyder, and his mentor, a slightly older professor of physics named J. Robert Oppenheimer.

The first paper described the mechanism of nuclear fission. Fission had been discovered nine months earlier by a team of physicists and chemists working in Berlin and Stockholm who found that bombarding uranium with neutrons could lead to a chain reaction with a startling release of energy. The basic reasons for the large release of energy in the process came from Einstein's famous equation, E = mc2, and were understood well. But a lot of questions remained: What was the general theory behind the process? Why did uranium split into two and not more fragments? Under what conditions would a uranium atom split? Would other elements also undergo fission?

Bohr and Wheeler answered many of these questions in their paper. Bohr had already come up with an enduring analogy for understanding the nucleus: that of a liquid drop that wobbles in all directions and is held together by surface tension until an external force that is violent enough tears it apart. But this is a classical view of the uranium nucleus. Niels Bohr had been a pioneer of quantum mechanics. From a quantum mechanical standpoint the uranium nucleus is both a particle and a wave represented as a wavefunction, a mathematical object whose manipulation allows us to calculate properties of the element. In their paper Wheeler and Bohr found that the uranium nucleus is almost perfectly poised on the cusp of classical and quantum mechanics, being described partly as a liquid drop and partly by a wavefunction. At twenty five pages the paper is a tour de force, and it paved the way for understanding many other features of fission that were critical to both peaceful and military uses of atomic energy.

The second paper, by Oppenheimer and Snyder, was not as long; only four pages. But these four pages were monumental in their importance because they described, for the first time in history, what we call black holes. The road to black holes had begun about ten years earlier when a young Indian physicist pondered the fate of white dwarfs on a long voyage by sea to England. At the ripe old age of nineteen, Subrahmanyan Chandrasekhar worked out that white dwarfs wouldn't be able to support themselves against gravity if their mass increased beyond a certain limit. A few years later in 1935, Chandrasekhar had a showdown with Arthur Eddington, one of the most famous astronomers in the world, who could not believe that nature could be so pathological as to permit gravitational collapse. Eddington was a previous revolutionary who had famously tested Einstein's theory of relativity and its prediction of starlight bending in 1919. By 1935 he had turned conservative.

Read more »