Tag: Hopf fibration

What Tangled Webs: The Hopf Fibration And Physics III

Posted on by Jochen Szangolies

by Jochen Szangolies

The Bloch sphere, the state space of a single qubit, as the Hopf fibration. To every point, a circle is associated which keeps track of the phase degree of freedom, according to the matching colors.

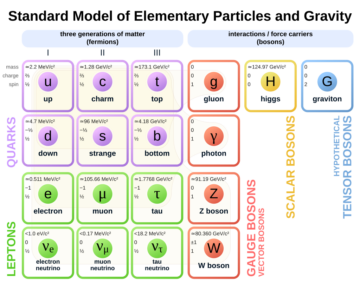
In the previous two installments of this series ([1], [2]), I have been engaged in the project of communicating a bit of the intuition behind the abstract notions of physics (and the necessary mathematics). My guiding principle in this attempt (essay in the literal sense) has been a famous quote of Hungarian mathematician and polymath John von Neumann: “In mathematics you don’t understand things. You just get used to them.”

This is an initially surprising notion. Mathematics, it seems, is the domain of pure intellect, where great minds wrestle with arcane concepts to squeeze droplets of eternal truth from Platonic realms of pure form. How does this square with ‘just getting used to it’?

I think that the poles of this apparent dichotomy are not as far removed as it might seem. The traditional view seems to emphasize singular mental effort, while von Neumann intimates, rather, repeated exposure—training, or diligent practice. The first is the province of what Daniel Kahnemann calls ‘System 2’, the effortful, explicit, step-by-step mode of reasoning that is often implicitly meant simply by ‘thought’. We analyze novel concepts, break them down into their components, resolve them into a step-by-step, algorithmic sequence, much like taking apart a watch to find what makes it tick.

But practice targets a different, implicit and automatic mode of thought: that of ‘System 1’, the associative, fast and heuristic mode of cogitation at work when you perform activities that require little explicit thought. Take riding a bike: there is no hope to learn it purely via the System 2-mode—that is, you can’t read a book on bike-riding, hop on and be off on your merry way. You need, rather, to train—to try, fail, and try again, until you get it right; and once you do, you’ll find yourself at a loss explaining exactly how. But after you have learned to do it, it ceases to become an explicit effort, instead happening apparently ‘by itself’, without you having to attend to the precise sequence of motions that keep your feet cranking the pedals, your arms turning the handles, and your entire body holding the balance.

Practice imparts an understanding that can’t be achieved by direct instruction. Getting used to something is a way to understand it that bypasses and complements the step-by-step process of analytical reason, a way to appreciate it at an intuitive Gestalt level rather than from the bottom up in terms of its individual components.

This is of course well appreciated by working physicists and mathematicians. But both popular science writing and popular culture at large paint a radically different picture. Read more »



Thursday, September 19, 2024

Wheels Within Wheels: The Hopf Fibration And Physics II

Posted on by Jochen Szangolies

by Jochen Szangolies

Crucial step in the proof that 1 + 1 = 2, coming on page 379 of Russell and Whitehead’s formalist tour-de-force Principia Mathematica.

In the last column, I have argued against the idea that understanding in mathematics and physics is transmitted via genius leaps of insight into obscure texts rife with definitions and abstract symbols. Rather, it is more like learning to cook: even if you have memorized the cookbook, your first soufflé might well fall in on itself. You need to experiment a little, get a feel for ingredients, temperatures, resting times and the way they interact before you get things right. Or take learning to ride a bike: the best textbook instructions won’t keep you from skinning your knees on your first try.

Practical skills are acquired through practice, and doing maths is just such a skill. However, mathematics (and physics by extension) may be unique in that it likes to pretend otherwise: that understanding is gleaned from definitions; that the manipulation of symbols on a page according to fixed rules is all there is to it. But no: just as you need an internal, intuitive model of yourself on a bike, its reactions to shifts in weight and ways to counteract developing instabilities, the skilled mathematician has an intuition of the mathematical objects under their study, and only later is that intuition cast into definitions and theorems.

At the risk of digressing too far, this is a general feature of human thought: we always start with an intuitive conception, only to later dress it up in formal garb to parade it before the judgment of others. We are not logical, but ‘analogical’ beings, our thoughts progressing as a series of dimly-grasped associations rather than crisp step-by-step derivations. If we do find ourselves engaged in the latter, then as a laborious, explicit, and slow ‘System 2’-exercise, rather than the intuitive leaps of ‘System 1’.

Indeed, it couldn’t be otherwise: how should we know whether a definition is accurate, if we didn’t have a grasp on the concept beyond that definition? Read more »

Tuesday, August 20, 2024

Music Of The Spheres: The Hopf Fibration And Physics

Posted on by Jochen Szangolies

by Jochen Szangolies

The particles of the Standard Model (and gravity). Image credit: Cush, CC0, via Wikimedia Commons

Modern physics in its full mathematical splendor introduces an array of unfamiliar concepts that daunt the initiate, and often even bewilder the pro (or is that just me?). A part of it is just that it’s a complex topic, and its objects of study are far removed from everyday experience: a quark or a black hole or a glueball is not something you’re likely to find on your desk. Well, maybe the latter, if you’ve been sloppy while crafting recently, but as so very often, physicists further confuse things by giving familiar names to unfamiliar concepts (spin, I’m looking your way).

But saying ‘it’s complicated’ is merely a fig leaf. Lots of things are complicated, and we manage to navigate them with ease. Many jobs involve reams of specialist knowledge, from plumbing to hedge-fond management, and even just navigating our webs of social relationships comes with considerable overhead. So what is it that makes physics special?

There is, of course, the already mentioned issue of the remoteness of its central concepts. Many of the complicated tasks we solve are so ingrained to us that we scarcely notice their complexity—the act of throwing a ball, or catching it out of thin air in flight, involves calculations that, in a realistic setting, stymied the efforts of robotics engineers for a long time. Likewise, the acquisition of language—even present-day Large Language Models (LLMs) still need to ‘read’ tens of trillions of words to acquire a degree of language fluency a human child can pick up just from what is spoken around them in their first couple of years. By comparison, an average reader would take something like 80.000 years of continuous reading time to ingest the text on which an LLM is trained!

These are tasks that, in some manner, are performed ‘natively’ by the human brain, without us noticing their complexity. Such tasks are sometimes classed as ‘System 1’-tasks in the dual-system psychology popularized by Daniel Kahnemann in his bestselling popular science book Thinking, Fast and Slow. In contrast, solving a mathematical equation or reasoning through a logic puzzle are step-by-step, explicit ‘System 2’-tasks you have to concentrate on—they’re not performed ‘by themselves’ the way catching a ball is. Read more »