by Jeroen Bouterse
 Have you ever been in this situation where you had to get a group of 3 men and their sisters across a river, but the boat only held two and you had to take precautions to ensure the women got across without being assaulted?
Have you ever been in this situation where you had to get a group of 3 men and their sisters across a river, but the boat only held two and you had to take precautions to ensure the women got across without being assaulted?
This problem is one of 53 puzzles in the oldest extant puzzle book in the Western (Latin) tradition: the Propositiones ad acuendos iuventes or problems to sharpen the young. Its authorship is uncertain but it is often and plausibly attributed to Alcuin, who possibly sent them to the Frankish ruler Charlemagne in 800 AD.[1] I hope you will allow me a brief introduction of these puzzles, before I go on to do what I hope will by then be redundant, namely spelling out why I think you should be thrilled by their existence.
Slugs and Pigeons
Alcuin’s puzzles are diverse, but puzzles of the same type often show up more than once. For example, some have us figure out a number based on a multiple of it, provided in a somewhat convoluted manner: a man seeing a certain number of horses and wishing that he possessed that number, and then that number again, and then a quarter more than that, for then he would possess a hundred horses (puzzle 4). There are questions that effectively ask how often a given area fits into another given area, how items can be distributed in discrete quantities given certain conditions, or how long it will take for one animal to overtake another or to cover a given distance. The first puzzle, for instance, has a leech invite a slug over for lunch, only to have us realize that it will take the poor creature centuries to get to its destination.
Puzzle 42 has the student add up all the numbers from 1 to 100. Or, to be more precise, figure out the number of pigeons on a staircase with 100 steps, where on the first step sits one pigeon, and every next step has one pigeon more than the previous. Which, admittedly, is way more fun than just adding numbers. Alcuin solves it by taking pairs of steps that together have 100 pigeons (such as the first and the 99th step). This gives 49 pairs, and leaves steps 50 and 100 unused, so that the sum is 4900 + 150 = 5050.[2]
The contexts for the questions range from the fanciful – as with the leech and the slug – to the mundane, as in a question how 204 eggs can be distributed equally among a group of monks. The context is sometimes ‘richer’ than the solution strictly requires – in the egg-distribution-question, for instance, we hear that the five priests get 85 eggs, the four deacons get 68, and the three readers get 51. Of course, this is much more information than we need.[3]
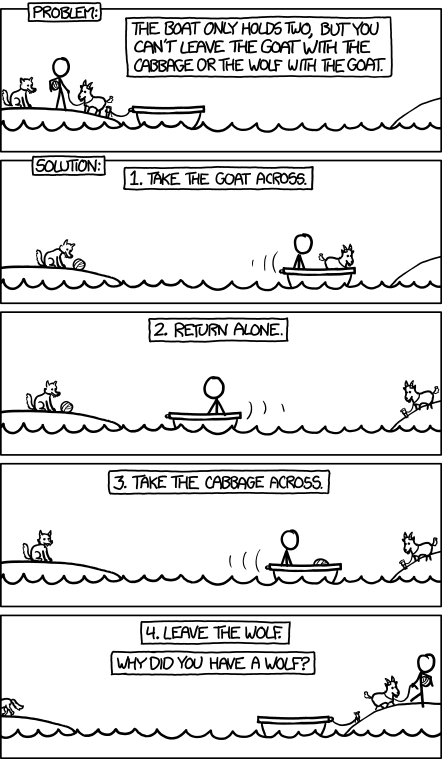
Three puzzles belong to the river-crossing genre.[4] One of these (17) is the one with the three men and their sisters. Another (19) involves a very heavy man and woman (each the weight of a loaded cart) and their two children, seeking to get across a river in a boat that can only just hold either of the adults or both of the children. A third (18) is most likely to be familiar to you: Alcuin’s text has the first known instance of the most famous river-crossing puzzle. Yes, that one. The one where you are responsible for a wolf, a goat and a cabbage, and have to row them across a river in a boat that only holds you and one of them, making sure never to leave the goat unsupervised with either the wolf or the cabbage. Maybe you were thinking about it right from the moment you started reading this article.
Encountering these riddles in an 8th-century collection gave me goosebumps. I knew of the existence of pre-modern mathematical puzzle books and had no real reason to be surprised that some of the most canonical riddles go back a long way – why wouldn’t they? Still, implicitly, I associate this genre with a historically recent ‘geek culture’ that revels in formalizing trivial situations and turning them into abstract brainteasers. The webcomic XKCD, for instance, refers to the wolf-goat-riddle to escalate a joke about the logistics of assigning designated drivers in a group. The recognition that the same brainteaser (or ‘sharpener’) commanded the attention and imagination of an 8th-century scholar felt, pardon the pathos, as a reminder of a common humanity: a universal appetite for puzzles that is apparently so pervasive that this meme could persist for centuries in traditions both oral and written.
Boat Size Then and Now
Of course, though the resemblance between Alcuin’s rendition of the puzzle and its typical modern version is remarkable, there are interesting differences. A small one is that Alcuin’s version does not involve just one cabbage but a bunch of cabbages. Slightly less elegant and economical, this strikes me as more realistic: don’t you also secretly think that if you found yourself in the single-cabbage situation, you would have found room in the boat for that one additional cabbage? Alcuin sensibly cuts off this response by inflicting a suitably large number of cabbages on us – I’m guessing a small boatload.
A more significant difference is that here as in other riddles, Alcuin does not spell out the rules of the game. He assumes that we have the common sense not to leave a wolf alone with a goat or a goat with a cabbage (let alone a bunch of cabbages). Less trivially, in riddle 17, he seems to take for granted that a woman is safe from assault when her brother is present, but that the presence of other women does not make a difference. Nowhere is this said out loud, but the stated solution of the riddle makes his assumption clear.
Alcuin may be aware that some of his situations are proxies to more abstract problems – his pigeons quite plainly stand for numbers – but his approach rarely encourages further abstraction and generalization. If he provides a method at all, rather than just a solution, it is usually a one-time trick.
By contrast, modern renditions of mathematical and logical puzzles are usually more explicit about their formal structure: a quick google search confirms that websites presenting the wolf-goat-cabbage puzzle will spell out for their readers when the wolf will eat the goat. Also, mathematicians have abstracted river-crossing puzzles into graph-theoretical and algebraic structures, studying generalized versions for their formal properties and proving their results.
This tendency towards generalization is not just a recent phenomenon, however. Raffaella Franci, a scholar who has traced Alcuin’s men-and-sisters-puzzle in the later Middle Ages (the male-female pairs then usually being husbands and wives), notes several 16th-century Italian authors who think about generalizing for the number of pairs. They usually insist on using a bigger boat.[5] Niccolò Tartaglia in his General trattato gives a solution for four pairs in a boat holding just two people, but this depends on a relaxation of the ‘jealousy condition’: it involves, for the briefest of moments, a woman being in proximity to another man without her husband. She immediately goes back, but Franci dryly remarks that the harm has been done: “authors with a more pessimistic attitude towards human nature do not permit such a situation and so this solution was considered wrong”.[6]
Leopards and Tigers
Tartaglia also describes the puzzle with the wolf, the goat and the cabbage. I already noted my (unjustified but no less genuine) surprise at realizing how apparently historically widespread the wolf-goat-cabbage riddle is. In fact, the Latin and Italian texts I have mentioned so far barely scratch the surface. The structure of the problem is so common that an authoritative school of folklorists has assigned it an index number in a classification of international folktales: it can be found in volume II of Hans-Jörg Uther’s Types of international folktales, as type (or ATU index number, named after Uther and his predecessors) 1579: ‘Carrying Wolf, Goat, and Cabbage across Stream’.[7] For the wolf we may substitute a lion or a jackal, and the cabbage may be exchanged for a bundle of hay or a pumpkin. Uther lists dozens of variants of the scene, mainly from Europe and Africa but also from Chinese or Mayan sources. There is a related entry, ‘ATU1579**’, that covers calculation puzzles like Alcuin’s 4th puzzle (mentioned above).
This folklorist approach provides a different perspective on river-crossing or other logical or mathematical riddles – as an instance not of mathematical or logical interest as such, but of tales involving clever men, having less in common perhaps with high school mathematics than with fairy tales, and with stories about how travelers manage to trick ogres and devils.
It also raises questions of influence or independent invention. In a 1990 article on African riddle-crossing puzzles, Marcia Ascher argues that differences in logical structure ought to count as evidence of independence: several African riddle-crossing versions are not just Alcuin’s riddle with a tiger, a sheep and a big spray of reeds (though these too exist), but a different riddle with formally different constraints.[8] A Swahili variant of the puzzle involves a situation where two out of the three creatures (a leopard, a goat and tree leaves that have to be delivered to the Sultan’s son) can be transported at once, but neither can be left alone with any other.
This requires a similar kind of out-of-the-box-thinking as the European variant: you will have to take one of the creatures back from the first trip, although now it doesn’t matter which one. It is this kind of cleverness that seems to be the common denominator of all of these puzzles. Not an interest in the abstract properties that lie behind their solutions, but a one-trick-at-a-time interest in any riddles, exercises and anecdotes that embody wit and intelligence, and help “sharpen” the mind – which, as you remember, is what Alcuin states he is after. This may be why every now and then, we will encounter a riddle whose solution is too cute to my taste. In puzzle 14, Alcuin asks us to figure out the number of footprints that a plowing ox leaves in the field at the end of the day, and tells us that since the ox precedes the plough, its footprints will not be left in the field. (Ugh.)
Even such rule-defying solutions can sometimes be delightful, however, as in one of Ascher’s examples:
“Still one more African version of the problem is found only among the Ila (Zambia) […]. The striking difference is that it involves four items to be transported: a leopard, a goat, a rat, and a basket of corn. The boat can hold just the man and one of these. This problem exemplifies the interrelationship of culture and logical constraints. After considering leaving behind the rat or leopard (and thus reducing the problem to one that can be solved logically), the man’s decision is that since both animals are to him as children, he will forego the river crossing and remain where he is!”[9]
This is, surely, the best of both worlds: it recognizes a problem to be close to a solvable one, but looks keenly and critically at the limitations of the solution.
Some solutions to river-crossing riddles, then, manage to combine abstraction and common sense. Well, XKCD never lets us down in that respect either. I will finish with its take on the puzzle:[10]
[1] ‘Problems to Sharpen the Young’, transl. John Hadley, comm. David Singmaster and John Hadley, Mathematical Gazette 76: 1992, 102-126: p. 103.
[2] This is a bit more haphazard than the method immortalized by an anecdote about the young Gauss (who simply took half the number of terms of the series and multiplied it by the sum of the first and the last term – a method also known before Alcuin’s time, for instance to the 5th-6th-century Indian mathematician Aryabatha). See the relevant part of the English translation of his Aryabhatiya: https://archive.org/stream/The_Aryabhatiya_of_Aryabhata_Clark_1930#page/n63/mode/2up ).
[3] According to medievalist Rutger Kramer, the text tries to drive home the point that in a monastery, everybody is supposed to be equal. ‘Ecce fabula!’ Problem-Solving by Numbers in the Carolingian World: The Case of the Propositiones ad Acuendos Iuvenes’ Proceedings of the 2015 MEMSA Student Conference, 2015, 15-40: p. 33.
[4] Actually, there is a fourth one (20), but its text is defective.
[5] Raffaella Franci, ‘Jealous Husbands Crossing the River: A Problem from Alcuin to Tartaglia’ in: Yvonne Dold-Samplonius, Doseph W. Dauben, Menso Folkerts, Benno van Dalen ed., From China to Paris: 2000 Years Transmission of Mathematical Ideas. Franz Steiner Verlag: Stuttgart 2002, p. 289-306: p. 295-296.
[6] Ibid., p. 299.
[7] Hans-Jörg Uther, The Types of International Folktales: A Classification and Bibliography, based on the System of Antti Aarne and Stith Thompson. Helsinki: Academia Scientiarum Fennica 2004. p. 318. Piret Voolaid, ‘Carrying a Wolf, a Goat, and a Cabbage across the Stream. Metamorphoses of ATU 1579’, Folklore: Electronic Journal of Folklore 35: 2007, 111–130. p. 114-115 has a rendition of Alcuin’s puzzle 19, which is also classified as belonging to the ATU 1579 type.
[8] Marcia Ascher, ‘A River-Crossing Problem in Cross-Cultural Perspective’, Mathematics Magazine 63: 1990, 26-29.
[9] Ascher, p. 28.
