Kevin Hartnett in Quanta Magazine:
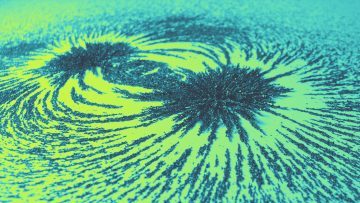
In the physical world, objects often push each other apart in an orderly way. Think of the symmetric designs formed by iron filings under the influence of a magnetic field, or the even spacing of parkgoers practicing social distancing.
When mathematicians look at the number line, they see the same type of trend. They look at the tick marks denoting the positive and negative counting numbers and sense a kind of numerical force holding them in that equal spacing. It’s as though, like mountain lions with their wide territories, integers can’t exist any closer together than 1 unit apart.
The spacing of the number line is the most basic example of a phenomenon found throughout the field of number theory. It crops up in the study of prime numbers and in the relationships between solutions to different types of equations. Mathematicians can better understand these important values by quantifying the force that acts between them.
“Things with number-theoretic significance tend to push each other apart. The name for this is a repulsion principle,” said Jacob Tsimerman of the University of Toronto. “We try to obtain repulsion principles and use them to get various other results.”
A lot of important work in number theory has to do with the way a repulsion principle influences polynomials, collections of coefficients and variables raised to powers. And for decades, mathematicians have been trying to pin down the exact magnitude of the repulsion in this setting.
In a proof posted online in late December, Vesselin Dimitrov of the University of Toronto finally did it.